Proving the veracity of the characteristic expression of the Pythagorean Theorem is a task as ancient as the discovery of the theorem itself. Today there are many ways to prove that the Pythagorean Theorem is completely valid, some of which are more famous than others.

In this post, we’ll discuss the most relevant ways used to prove that the Pythagorean Theorem is correct.

Pythagoras’ proof of the Pythagorean Theorem

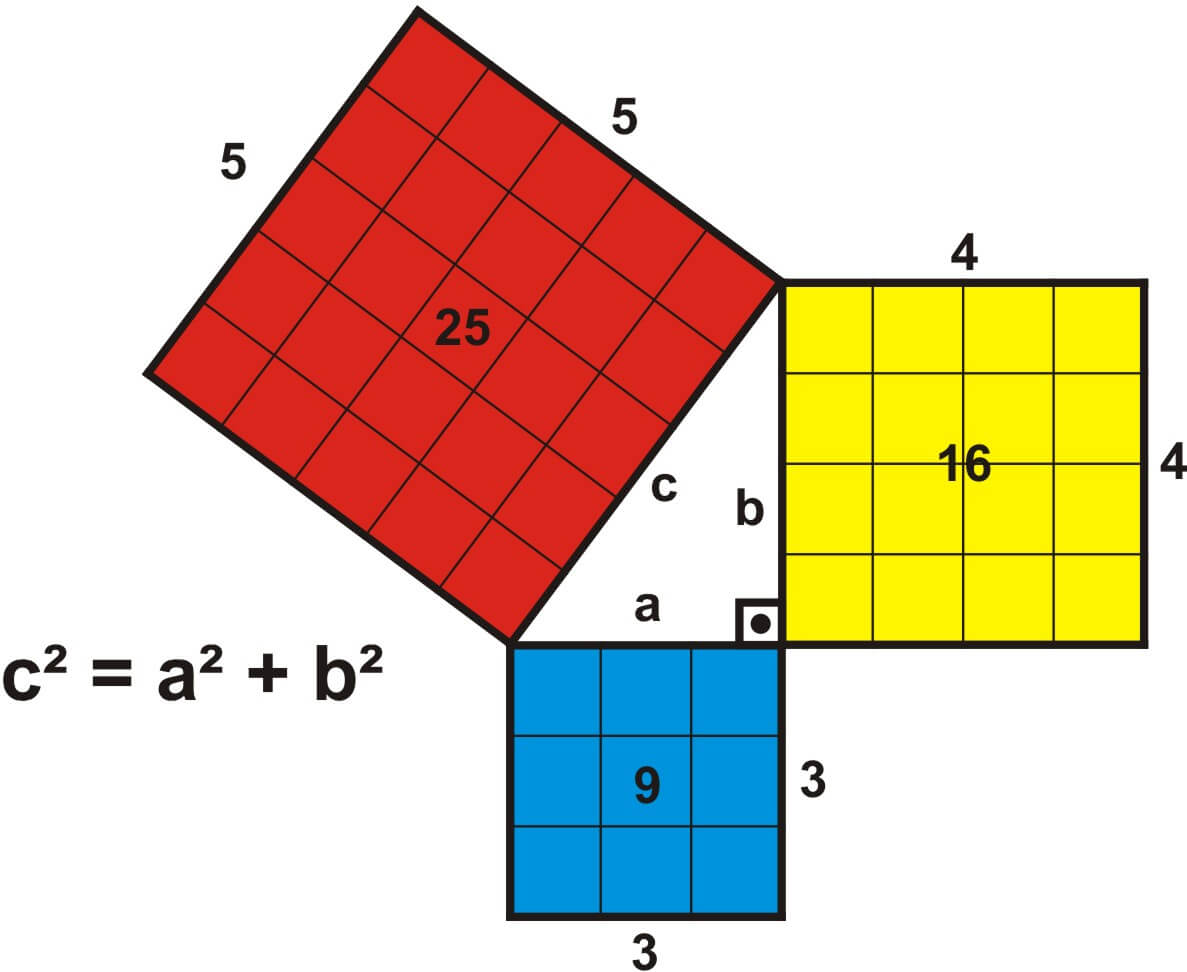
Pythagoras himself presented the most famous proof of this theorem which shows the equality using the equality of the areas created by the lengths of the sides of a right triangle.

Euclid’s proof of the Pythagorean Theorem

Proposition I.47 in Euclid’s Elements mentions the Pythagorean Theorem and proves it by making the corresponding area for the sides of the triangle. So if we draw a straight line perpendicular to the hypotenuse that passes through the right angle, in addition to having 3 squares with sides that are the same size as each side of the triangle and which are adjacent to them, we also see that the area of each square corresponding to the two legs is equal to the corresponding division we made by drawing a line through the square of the hypotenuse.

Plato’s proof of the Pythagorean Theorem

Plato’s Dialogues is a famous compendium which includes a proof of the Pythagorean Theorem based on a triangle whose non-right angles measure 45°; in other words, a right triangle that is also isosceles.

There are also empirical proofs whose calculations are based in different everyday situations, like the use of water to confirm the veracity of the proof of the Pythagorean Theorem proposed by Euclid or the cutting of geometric shapes that are used as puzzles and thanks to which we can prove the Pythagorean Theorem.